Vittore Cossalter - Translation: Tom Timmermans

Vittore Cossalter - Translation: Tom Timmermans

The gyroscopic effect originates when the wheel, performing a rotational movement around its own axis with velocity w, is also brought into rotation around a second axis, perpendicular to the former with velocity W.

( w = constant; W= constant; momento giroscopico=gyroscopic momentum; velocità angolare di rotazione della ruota=angular velocity of wheel rotation)
The gyroscopic effect turns up as a couple acting around an axis perpendicnlar to the preceding axes. The value of the gyroscopic moment equals the product of the polar moment of inertia of the wheel and the rotational velocities w and W .
In motorcycle dynamics, various gyroscopic moments are in control:
Gyroscopic effects from yaw motion
Let us consider a front or a rear wheel, turning around its own axis at constant velocity w>, while the motorcycle performs a curve with radius R, likewise at constant yaw velocity W.
The movement of the wheel into the curve generates a gyroscopic moment around the horizontal axis, tending to raise up the motorcycle:
![]()
>where I indicates the polar inertial moment of the wheel with respect to its own axis, w the rotational velocity around the same axis,W the rotational yaw velocity, equal to the ratio of the forward velocity of the motorcycle to the radius of the curve.
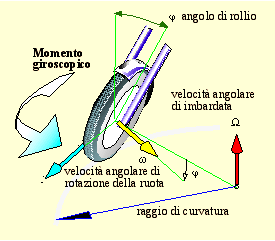
( angolo di rollio=roll angle; momento giroscopico=gyroscopic moment; velocità angolare di imbardata=angular yaw velocity; velocità angolare di rotazione della ruota=wheel rotation angular velocity; raggio di curvatura=radius of curvature)
Now, let us examine the effect of both wheels.
The gyroscopic moment results:
![]()
where If and Ir indicate the moments of inertia of the front and rear wheel. If we suppose the gyroscopic effect to be zero and also ignore the effect attributed to tyre thickness, the equilibrium condition for steady motion in the curve (forward velocity and radius of curvature all constant) imposes that the resultant of the weight force and the centrifugal force intersects the line connecting the contact points of both wheels. In this ideal case the motorcycle's roll angle issues from the simple relation:
![]()
The gyroscopic effect contributes to a greater roll inclination with respect to that ideal case, calculated considering the gyroscopic effect to be zero.
>
( angolo di rollio=roll angle; forza d'inerzia centrifuga=centrifugal inertial force; baricentro=centre of gravity; forza peso=weight force; velocità angolaredi imbardata=angular yaw velocity; velocità angolare di rotazione della ruota=wheel rotation angular velocity; raggio di curvatura=radius of curvature)
The increase Dj in roll angle, due to the gyroscopic effect, does not facilitate the motorcycle's manoeuvrability, because the motorcycle, in order to attain the steady roll angle (which is more elevated), needs more time.
Gyroscopic effects from roll motion.
Gyroscopic effect on the handlebars (front wheel roll).
This gyroscopic effect occurs when the motorcycle inclines.
 |
 |
where e indicates the inclination angle of the handlebars. The gyroscopic moment therefore tends to rotate the handlebars to the right, that is, facilitates entering the motorcycle into the curve (indeed, the increase in steering angle causes a reduction of the radius of curvature). Similarly, when the rolling motion changes sign and the motorcycle returns to the vertical position, the gyroscopic moment tends to diminish the steering angle, facilitating then the transition from motion in curve to straight line motion. Therefore, one can state that this gyroscopic effect has a stabilizing influence, in this way that it tends to maintain the motorcycle in equilibrium by exerting an aligning action.
Global gyroscopic effect on the motorcycle.
Let us examine again a rolling motion from left to right: the gyroscopic moment acting on the motorcycle equals:
![]()
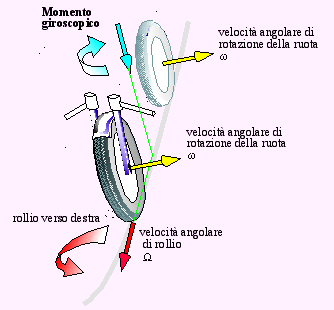
>(figura: gyroscopic moment; angular velocity of wheel rotation; angular velocity of wheel rotation; roll to the right; angular roll velocity )
During the roll phase, the gyroscopic effect from the motion of the wheels generates a moment, tending to yaw the vehicle, as demonstrated in the figure.
Gyroscopic effects from handlebar motion.
Since the wheel's direction of motion is perpendicular to the steering axis, turning the handlebars from right to left generates a gyroscopic moment around the roll axis:
![]()
represents the inclination angle of the handlebars.
 |
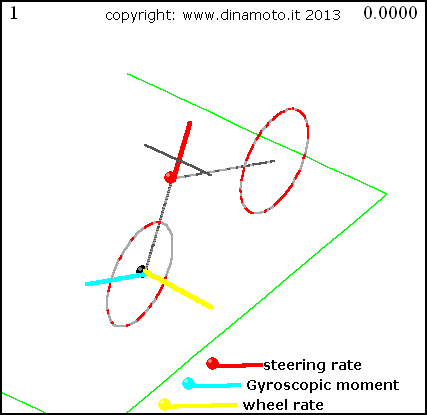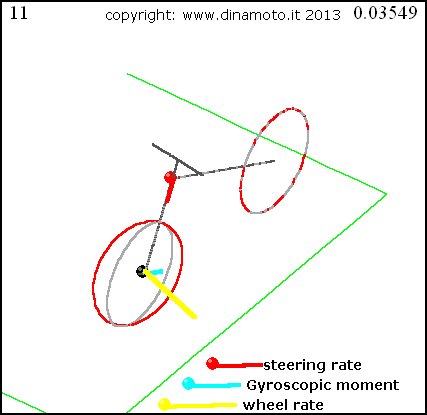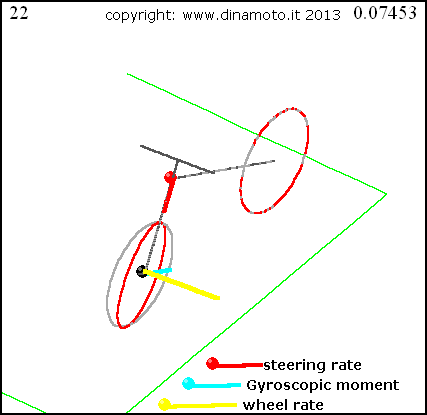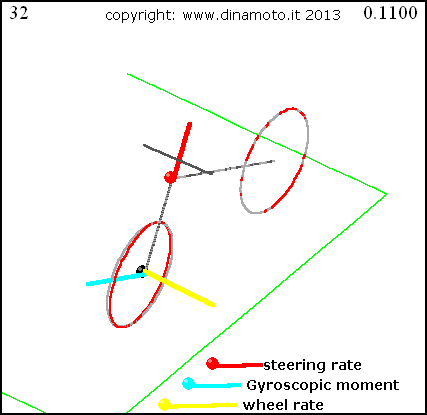 |
This moment tends to tilt the motorcycle in the opposite direction from the steering motion; for this reason, to curve into a direction during fast driving, the rider exerts a quick rotation of the handlebars in the opposite direction. The higher the velocity of handlebar rotation W, the higher will be the moment that tends to incline the motorcycle into the opposite direction.
For further reading: V. Cossalter Motorcycle dynamics.