Shaking forces of twin engines

Examples

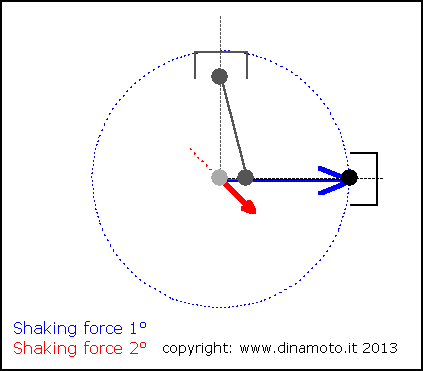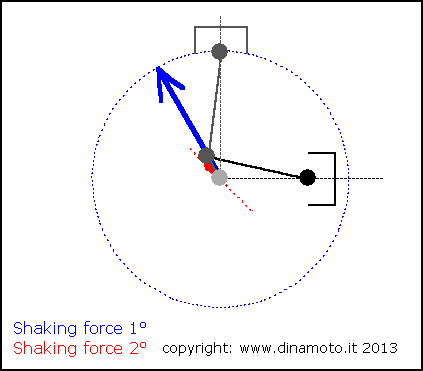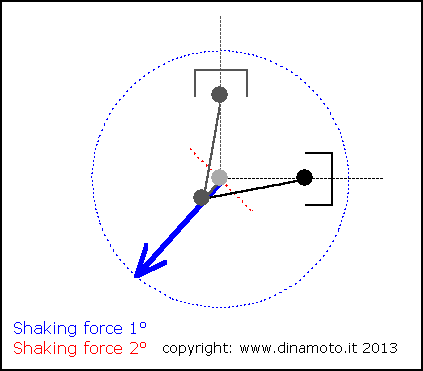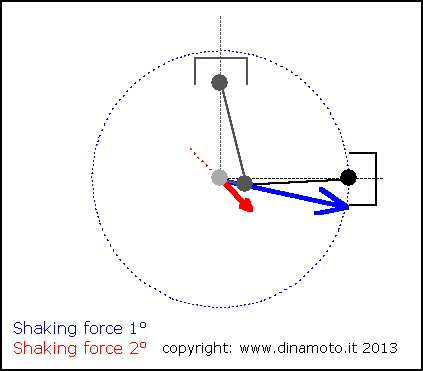
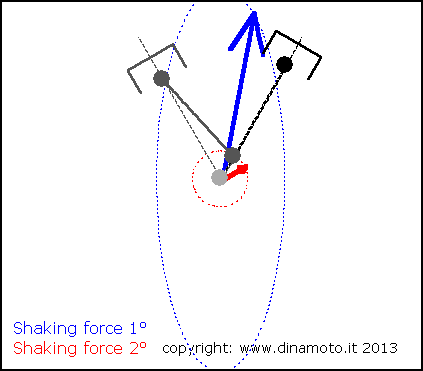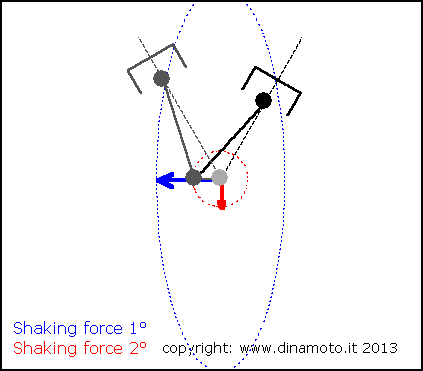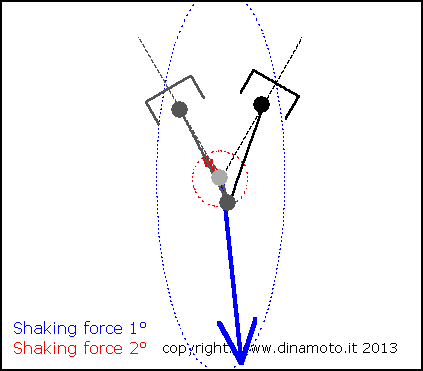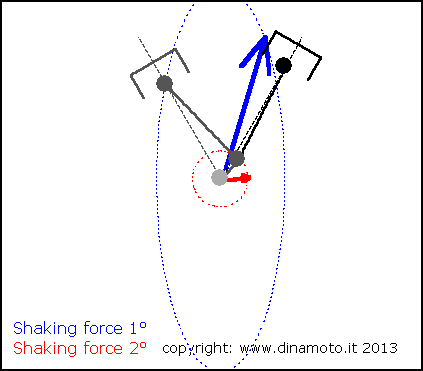
In the following examples, theblue arrow represents the first inertia force, and the red one the second inertia force
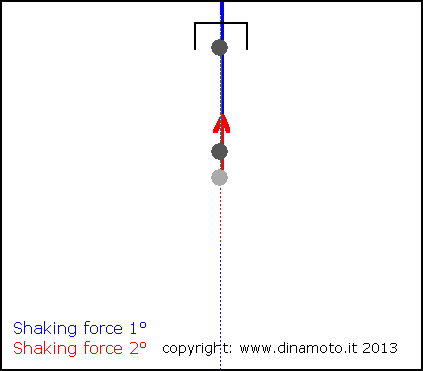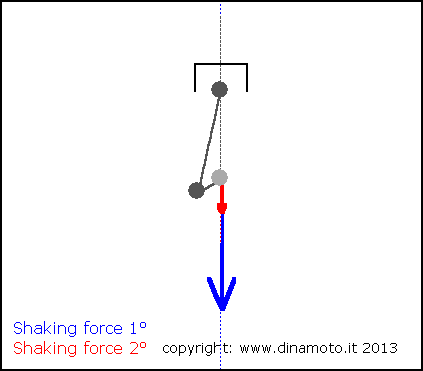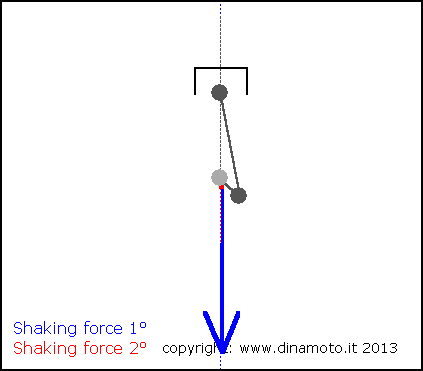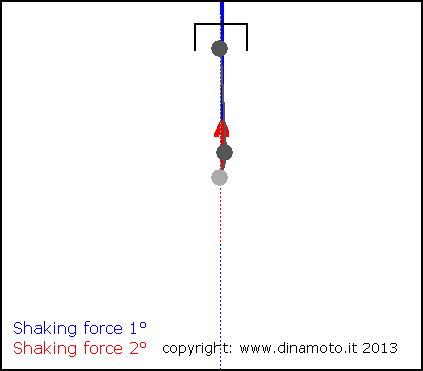
0 =degree cylinders angle, 0 =degree crankshaft angle
( Norton Comando)
90 =degree cylinders angle, 0 =degree crankshaft angle
(Moto Guzzi, Ducati)
45 =degree cylinders angle, 0 =degree crankshaft angle
(Harley-Davidson)
60 =degree cylinders angle,0 =degree crankshaft angle
( Aprilia 1000 RSV, this model has two countershafts to balance the first order force and the moment generated)
52 =degree cylinders angle, -76 =degree crankshaft angle
( Honda Transalp)
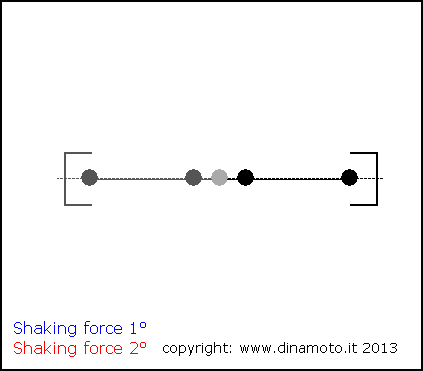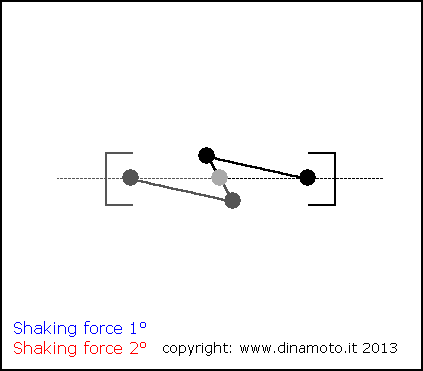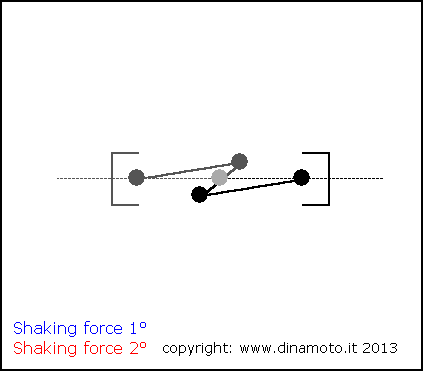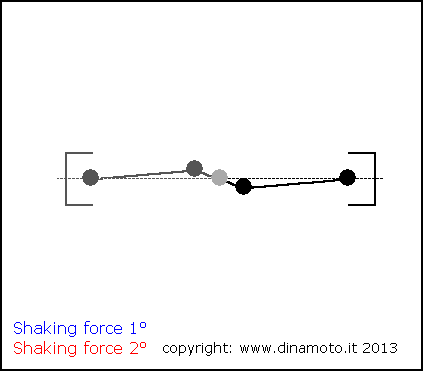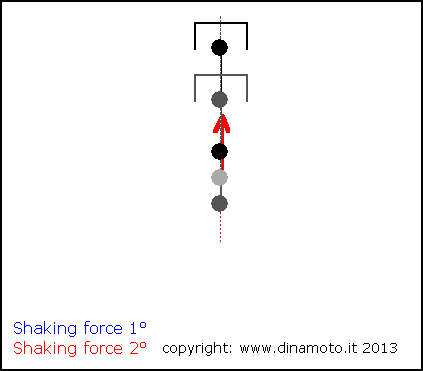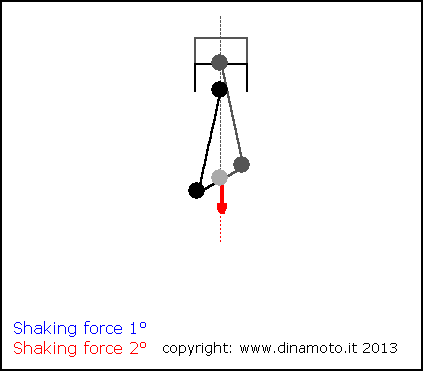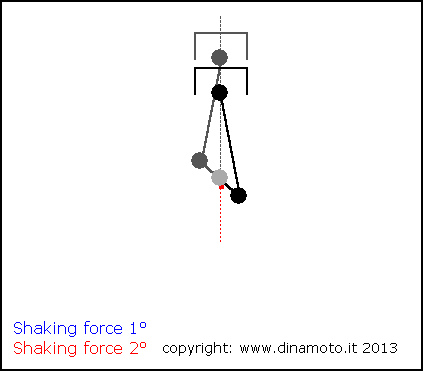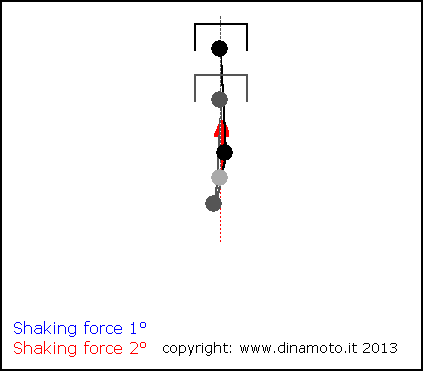180 =degree cylinders angle, 180 =degree crankshaft angle
( BMW boxer)
0 =degree cylinders angle,270 =degree crankshaft angle
( Yamaha TDM 850)

And now the theory

Let us consider the schematic drawing of a twin engine as shown in the figure


Fig. 1 Scheme of twin engines and shaking forces

As is well known, the shaking forces are the following:

dove:
mC = reciprocating mass located at the wrist pin, composed of the piston mass and the portion of the mass of the connecting-rod which is assumed to be concentrated at the wrist pin (about one-third ;
w =angular velocity;
r = crank radius;
b =connecting rod length;
I b= residual inertial moment of the connecting-rod ( negligible);

The components of the primary inertia force (first order) are:

It should be noted that the forces Fn re extremely reduced if compared with primary and secondary inertia forces.
This force rotates in the same direction as that of the cranks and traces an ellipsis or a circle in the x-y plane, depending on the amplitudes and phases of the two components.
Complete balancing is possible only if the two components have the same amplitudes and a phase angle equal to 90°. To reach this condition, the angle between the two cranks must respect this equation:
-

In this case, the simple addition of a mass in opposition to the primary inertial force is enough to obtain a complete balance. If the polar diagram of the primary force is an elliptical curve, the balance is only partial.
The components of the secondary inertia forces are the following:

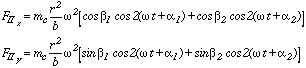
These components represent a force, generally with non-constant amplitude, rotating with a velocity double that of the cranks.
The primary and secondary forces are not in the same plane. For this reason, unbalanced couples will be set up which tend to rotate the engine around an axis perpendicular to the z axis. The values of these couples can be determined by multiplying the force by the distance between the two cylinders

wordpress visitor counter